In this unit, you will learn about slope, slope as a rate of change, direct variation, and partial variation.

First, we learned about slope. Slope is the steepness of a line, and it is measured by its slope. The slope of the straight line is the ratio of the rise to the run between both points.

The equation for slope is m = rise/run (rise over run), where m is slope.

Therefore, horizontal lines have a slope of 0 (no rise), and vertical lines have a slope that is undefined (no run, cannot divide by zero).

 Another thing we learned was slope as a rate of change. A rate of change compares the change in one quantity relative to a change in another quantity.
Unlike slope, though, a rate of change is expressed in units! For example, km/h.

Basically, slope and rate of change are the same thing.

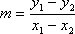The formula for rate of change is the change in dependent variable divided by the change in independent variable. So, the formula would be y2 - y1/ x2 - x1.

EXAMPLES: Loujain runs 25 km in 5 hours. What is her speed?
 = 25/5
 =5km/h

Sarah has a race with Sohyun. Sarah ran 30km in 3 hours, and Sohyun ran 36km in 6 hours. Who ran more?
Sarah-  =30/3
            =10 km/h

Sohyun-  = 36/6
              = 6 km/h
Sarah ran more.

Direct variation is the relationship between 2 variables in which one variable is a constant multiple of the other. Some characteristics of direct variation is how the graph will always be linear (straight line), the graph will always intercept the y-axis at the origin, and the equation for direct variation is always y = mx.

EXAMPLE: y=2x
                   D=5t
                   C=25h
                  H=10t

Partial variation is when the relationship between two variables have both a fixed and variable amount. Some characteristics of partial variation is how the fixed variable does not change- although the variable value changes, the graph intersects below or above the y-axis, the equation is always y = mx + b, and the line on a partial variation graph never passes through the origin.

EXAMPLE: C = 2.00 + 0.50n
                    T = 232 + 3n
                    y = 3.5x + 3
                    y = 0.2x + 5
TIP: the m (slope) and b (fixed cost) are constants.
m represents the constant of variation (multiple).
b represents the fixed, initial value.

Labels: Lessons

Hello, my name is Sohyun Kim, and I am in grade 9. This site is for my math summative project, so no hateful comments please. I generally like to read, draw, read manga, and watch anime in my spare time.
Normal | Bold | Strikeout | Italic | Underline | Link | big | small

Polynomials: Algebraic expressions made up of terms
Monomial: 1 term
Binomial: 2 terms
Trinomial: 3 terms
Terms: Made up of a coefficient and variable(s)
Power: Product of identical factors and consists of 2 parts- exponent and base. Coefficient: ----
Variable: ----
Constant/constant term: A term with no variable
Hypothesis: A theory or statement that is either true or false
Statistics: The collection, organization, and analysis of numerical data
Primary Data: Data that comes from experiments and surveys done by researchers
Secondary Data: Data that already exists- comes from sources such as the internet, magazines, and Statistics Canada
Interpolation: Prediction involving a point within the set of data
Extrapolation: Prediction involving a point outside the set of data
Linear Relation: Relation between 2 variables, forms a straight line
Non-Linear Relation: Relation between 2 variables, does NOT form a straight line
Curve of best fit: Curve that comes closest to the points of a non-linear relation
First Differences: -----
Slope: The steepness of a line- ratio of the rise to the run between both points
Direct Variation: A relationship between 2 variables in which one variable is a constant multiple of the other
Partial Variation: When a relationship between 2 variables have both a fixed and variable amount
Continued -HERE-

Polynomials / Equations / Relations / Linear Relations / Analytic Geometry / Measurement / Helpful Tips /